Cassie and Mayr Arc Models for a Circuit Breaker
This example shows the use of the Cassie and Mayr arc model for simulating high voltage circuit breaker interruption
P.H. Schavemaker, Power Systems Laboratory, Delft University of Technology, the Netherlands. P.H.Schavemaker@its.tudelft.nl
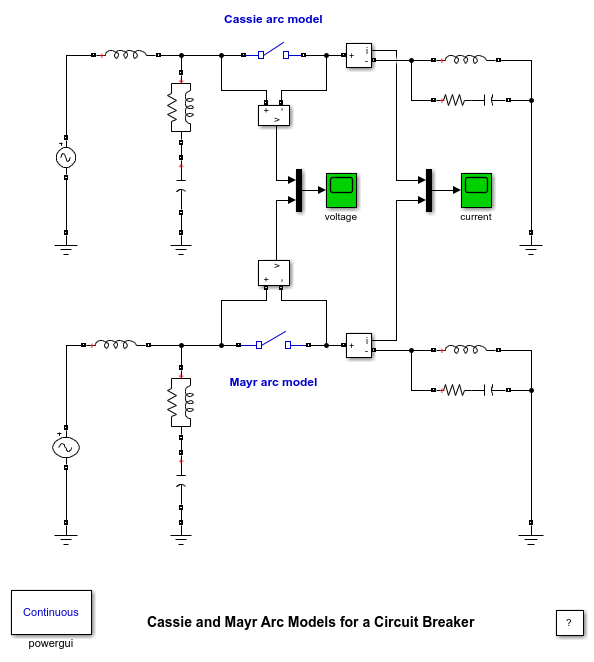
Description
Two identical circuits are displayed: one with a Cassie and one with a Mayr arc model. The circuit is a simple representation of a circuit breaker interrupting a short-line fault. At the source side of the circuit breaker a circuit is present for reproducing a (2-parameter IEC) transient recovery voltage, while the RLC circuit at the line side represents a short transmission line that is short circuited.
A stiff solver is recommended for solving this problems because of the nonlinear behavior of the arc.
Simulation
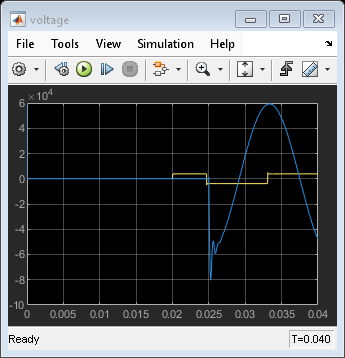
Figure 2: Voltage comparisons for the Cassie and Mayr arc models.

Figure 3: Current comparisons for the Cassie and Mayr arc models.
The following observations become clear from the simulation: The arc behaves as a non-linear resistance; therefore both arc current and voltage pass the zero value at the same time. As the power input into the arc channel is zero at that time, the current zero crossing is the place where the interruption takes place.
The Cassie arc model has a constant arc voltage in the high current interval, while the Mayr arc model shows an increasing arc voltage (the extinction peak) close to the current zero crossing.
The Cassie arc model fails to interrupt the short circuit current, while the Mayr arc model interrupts and shows a small post-arc current of 0.6A immediately after the current zero crossing. This current is caused by the transient recovery voltage building up over the non-infinite arc resistance.
In case of the Mayr arc model (the successful interruption), the transient recovery voltage (TRV) builds up over the breaker. This voltage consists of the 2-parameter TRV and the high frequency voltage oscillation of the line side, which you can observe by zooming in.
Additional Notes
This model cannot be run in accelerator mode due to modeling constraints.
