使用模型对象进行控制系统建模

模型对象可以表示控制架构的单个组件，如被控对象、作动器、传感器或控制器。您可以连接模型对象来构建模块图的聚合模型以表示多个元素的组合响应。

例如，以下控制系统包含一个预滤波器 F、一个被控对象 G 和一个控制器 C，它们以单环配置排列。该模型还包括一个传感器动态特性表示 S。

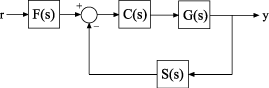
您可以将每个组件表示为一个模型对象。您不需要为每个组件使用相同类型的模型对象。例如，将被控对象 G 表示为零极点增益 (zpk) 模型，它在 s = -1 处有一个双极点；将 C 表示为 PID 控制器，将 F 和 S 表示为传递函数：

G = zpk([],[-1,-1],1);
C = pid(2,1.3,0.3,0.5);
S = tf(5,[1 4]);
F = tf(1,[1 1]);

然后，您可以将这些元素结合起来，构建表示您的控制系统或整个控制系统的模型。例如，创建开环响应 SGC：

open_loop = S*G*C;

要构建未滤波闭环响应的模型，请使用 feedback 命令：

T = feedback(G*C,S);

要对从 r 到 y 的整个闭环系统响应进行建模，请将 T 与滤波器传递函数结合使用：

Try = T*F;

结果 open_loop、T 和 Try 也是线性模型对象。您可以使用 Control System Toolbox™ 控制设计和分析命令对其进行操作。例如，绘制整个系统的阶跃响应：

stepplot(Try)

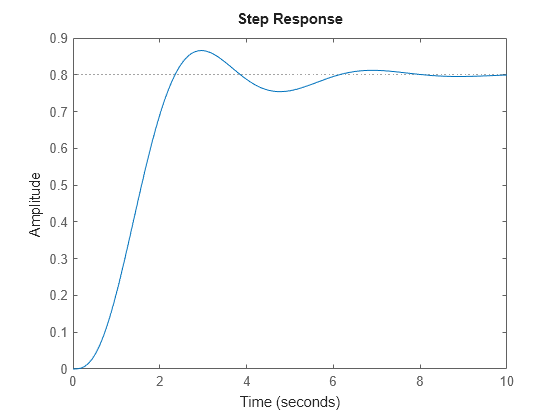
MATLAB figure

当您组合各数值 LTI 模型时，生成的数值 LTI 模型表示聚合系统。生成的模型不保留来自组合组件的原始数据。例如，T 不会单独跟踪组合在一起来创建 T 的组件 G、C 和 S 的动态特性。

另请参阅