Test, Visualize, and Enforce Passivity of Rational Output

This example shows how to test, visualize, and enforce the passivity of output from the rational function.

S-Parameter Data Passivity

Time-domain analysis and simulation depend critically on the ability to convert frequency-domain S-parameter data into causal, stable, and passive time-domain representations. Because the rational function guarantees that all poles are in the left half plane, rational output is both stable and causal by construction. The problem is passivity.

N-port S-parameter data represents a frequency-dependent transfer function H(f). You can create an S-parameters object in RF Toolbox™ by reading a Touchstone® file, such as passive.s2p, into the sparameters function.

You can use the ispassive function to check the passivity of the S-parameter data, and the passivity function to plot the 2-norm of the N-by-N matrices H(f) at each data frequency.

S = sparameters('passive.s2p');
ispassive(S)

ans = logical
   1

passivity(S)

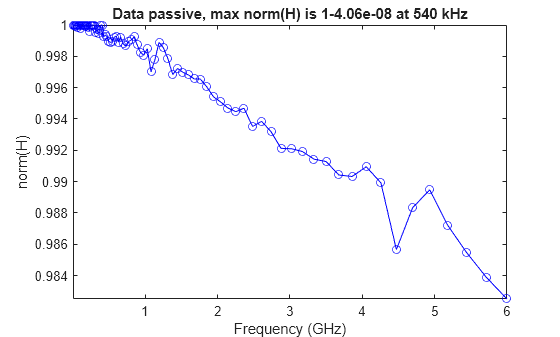
Figure contains an axes object. The axes object with title Data passive, max norm(H) is 1-4.06e-08 at 540 kHz, xlabel Frequency (GHz), ylabel norm(H) contains an object of type line.

Testing and Visualizing `rational` Output Passivity

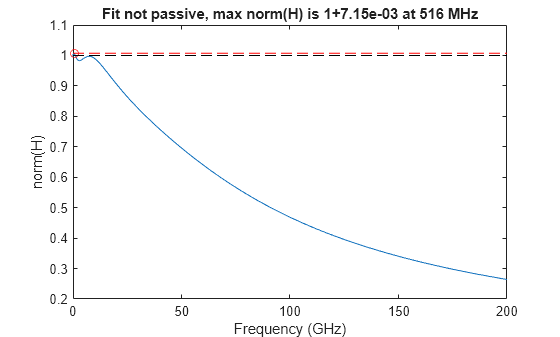
The rational function converts N-port S-parameter data, S, into an object that represents a rational fit to the data. Using the ispassive function on the output reports that even if input data S is passive, the output fit is not passive. In other words, the norm H(f) is greater than 1 at some frequency in the range [0,Inf].

The passivity function takes the output of the rational function as input and plots its passivity. This is a plot of the upper bound of the norm(H(f)) on [0,Inf], also known as the H-infinity norm.

fit = rational(S);
ispassive(fit)

ans = logical
   0

passivity(fit)

Figure contains an axes object. The axes object with title Fit not passive, max norm(H) is 1+7.15e-03 at 516 MHz, xlabel Frequency (GHz), ylabel norm(H) contains 4 objects of type line. One or more of the lines displays its values using only markers

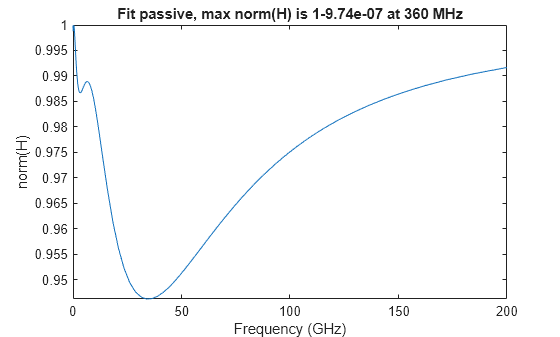
The makepassive function takes as input a rational object, and produces a passive fit by using convex optimization techniques to optimally match the data of the S-parameter input S while satisfying passivity constraints. The residues and direct term of the output pfit are modified, but the poles of the output pfit are identical to the poles of the input fit.

pfit = makepassive(fit,'Display','on');

Iter	H-infinity norm	Frequency	Error (dB)	Constraints
0		1+7.15e-03		516 MHz		-40.4597
1		1+5.44e-04		319 MHz		-41.7548	5
2		1+7.83e-05		383 MHz		-41.7319	7
3		1+7.93e-06		360 MHz		 -41.728	9
4		1-9.00e-07		359 MHz		-41.7273	10

ispassive(pfit)

ans = logical
   1

passivity(pfit)

Figure contains an axes object. The axes object with title Fit passive, max norm(H) is 1-9.00e-07 at 359 MHz, xlabel Frequency (GHz), ylabel norm(H) contains an object of type line.

all(pfit.Poles == fit.Poles)

ans = logical
   1

Generate Equivalent SPICE Circuit from Passive Fit

The generateSPICE function takes a passive fit and generates an equivalent circuit as a SPICE subcircuit file. The input fit is an object as returned by rational with an S-parameters object as input. The generated file is a SPICE model constructed solely of passive R, L, C elements and controlled source elements E, F, G, and H.

generateSPICE(pfit,'mypassive.ckt')
type mypassive.ckt

* Equivalent circuit model for mypassive.ckt
.SUBCKT mypassive po1 po2
Vsp1 po1 p1 0
Vsr1 p1 pr1 0
Rp1 pr1 0 50
Ru1 u1 0 50
Fr1 u1 0 Vsr1 -1
Fu1 u1 0 Vsp1 -1
Ry1 y1 0 1
Gy1 p1 0 y1 0 -0.02
Vsp2 po2 p2 0
Vsr2 p2 pr2 0
Rp2 pr2 0 50
Ru2 u2 0 50
Fr2 u2 0 Vsr2 -1
Fu2 u2 0 Vsp2 -1
Ry2 y2 0 1
Gy2 p2 0 y2 0 -0.02
Rx1 x1 0 1
Fxc1_2 x1 0 Vx2 1.73983662941813
Cx1 x1 xm1 4.40797252381963e-09
Vx1 xm1 0 0
Gx1_1 x1 0 u1 0 -0.0637780116111523
Rx2 x2 0 1
Fxc2_1 x2 0 Vx1 -1.10434906482951
Cx2 x2 xm2 4.40797252381963e-09
Vx2 xm2 0 0
Gx2_1 x2 0 u1 0 0.0704331874794615
Rx3 x3 0 1
Cx3 x3 0 2.46549218243635e-12
Gx3_1 x3 0 u1 0 -0.857110973445692
Rx4 x4 0 1
Cx4 x4 0 1.19630314783494e-11
Gx4_1 x4 0 u1 0 -2.76936140494346
Rx5 x5 0 1
Cx5 x5 0 1.85575923283012e-11
Gx5_1 x5 0 u1 0 -1.31122037346803
Rx6 x6 0 1
Cx6 x6 0 8.71015575423206e-11
Gx6_1 x6 0 u1 0 -0.691518683335449
Rx7 x7 0 1
Cx7 x7 0 5.76402180507914e-10
Gx7_1 x7 0 u1 0 -0.0721137010476538
Rx8 x8 0 1
Cx8 x8 0 1.32870599427364e-08
Gx8_1 x8 0 u1 0 -0.853443666992151
Rx9 x9 0 1
Fxc9_10 x9 0 Vx10 1.74899789209539
Cx9 x9 xm9 4.40797252381963e-09
Vx9 xm9 0 0
Gx9_2 x9 0 u2 0 -0.0646435567444553
Rx10 x10 0 1
Fxc10_9 x10 0 Vx9 -1.09856447702868
Cx10 x10 xm10 4.40797252381963e-09
Vx10 xm10 0 0
Gx10_2 x10 0 u2 0 0.0710151151082463
Rx11 x11 0 1
Cx11 x11 0 2.46549218243635e-12
Gx11_2 x11 0 u2 0 -0.903013298701984
Rx12 x12 0 1
Cx12 x12 0 1.19630314783494e-11
Gx12_2 x12 0 u2 0 -2.78912399833068
Rx13 x13 0 1
Cx13 x13 0 1.85575923283012e-11
Gx13_2 x13 0 u2 0 -1.32139928022124
Rx14 x14 0 1
Cx14 x14 0 8.71015575423206e-11
Gx14_2 x14 0 u2 0 -0.731804932668761
Rx15 x15 0 1
Cx15 x15 0 5.76402180507914e-10
Gx15_2 x15 0 u2 0 -0.0762155756410513
Rx16 x16 0 1
Cx16 x16 0 1.32870599427364e-08
Gx16_2 x16 0 u2 0 -0.853738421522371
Gyc1_1 y1 0 x1 0 -1
Gyc1_2 y1 0 x2 0 -1
Gyc1_3 y1 0 x3 0 -1
Gyc1_4 y1 0 x4 0 0.133812501038142
Gyc1_5 y1 0 x5 0 -0.577025534598742
Gyc1_6 y1 0 x6 0 -1
Gyc1_7 y1 0 x7 0 1
Gyc1_8 y1 0 x8 0 0.998930222960295
Gyc1_9 y1 0 x9 0 0.9812580927411
Gyc1_10 y1 0 x10 0 0.985349183222844
Gyc1_11 y1 0 x11 0 0.820751465966825
Gyc1_12 y1 0 x12 0 -1
Gyc1_13 y1 0 x13 0 1
Gyc1_14 y1 0 x14 0 0.920287412225175
Gyc1_15 y1 0 x15 0 -0.91669216502865
Gyc1_16 y1 0 x16 0 -1
Gyd1_1 y1 0 u1 0 0.999298520305084
Gyd1_2 y1 0 u2 0 -0.0123008134989598
Gyc2_1 y2 0 x1 0 0.992716554851763
Gyc2_2 y2 0 x2 0 0.990345122187567
Gyc2_3 y2 0 x3 0 0.823596484403637
Gyc2_4 y2 0 x4 0 -1
Gyc2_5 y2 0 x5 0 1
Gyc2_6 y2 0 x6 0 0.974339278618892
Gyc2_7 y2 0 x7 0 -0.968808354500153
Gyc2_8 y2 0 x8 0 -1
Gyc2_9 y2 0 x9 0 -1
Gyc2_10 y2 0 x10 0 -1
Gyc2_11 y2 0 x11 0 -1
Gyc2_12 y2 0 x12 0 0.207629903960971
Gyc2_13 y2 0 x13 0 -0.668109166178573
Gyc2_14 y2 0 x14 0 -1
Gyc2_15 y2 0 x15 0 1
Gyc2_16 y2 0 x16 0 0.998693355982142
Gyd2_1 y2 0 u1 0 0.0129572556240674
Gyd2_2 y2 0 u2 0 0.999452705396658
.ENDS

Test, Visualize, and Enforce Passivity of Rational Output

S-Parameter Data Passivity

Testing and Visualizing rational Output Passivity

Generate Equivalent SPICE Circuit from Passive Fit

Related Topics

Testing and Visualizing `rational` Output Passivity