EIRP

EIRP is the amount of power that would have to be radiated by an isotropic antenna to produce the equivalent power from the actual antenna in the direction with highest antenna gain.

EIRP is the total radiated power (P_t) from a transmitter antenna times the antenna gain (G_t).

EIRP = P_t ✕ G_t

If expressed in decibels:

EIRP (dBm) = P_t (dBm) + G_t (dBi)

The EIRP enables comparisons between different emitters regardless of type, size, or form.

Considering different losses such as feeder loss L_f and pointing loss L_p into account:

EIRP (dBm) = P_t (dBm) + G_t (dBi) - L_f - L_p (dB)

This plot shows an isotropic antenna 2-D radiation pattern for all azimuth angles at 0 degrees elevation.

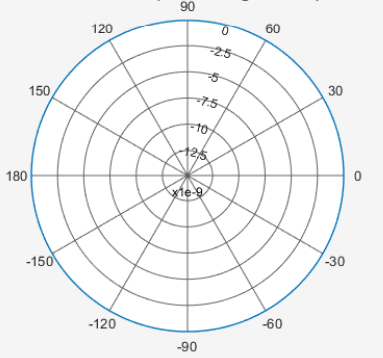
This plot shows a directional helix antenna 2-D radiation pattern with a main lobe, a back lobe, and side lobes.

Feeder Loss

This loss occurs in several components between the antenna and the receiver or the transmitter device, such as couplers, filters, and waveguides. Feeder loss is due to the resistance and imperfections in the transmission line material, which causes part of the signal energy to be dissipated as heat.

On the transmitting end, feeder losses are typically a part of the output of the high power amplifier (HPA) and the radiating antenna. Similarly, on the receiving end, feeder losses are typically a part of the output of the antenna to the input of the low noise amplifier.

FSPL

FSPL refers to the spread of the signal through space, which results in loss of the strength of the signal. Calculate FSPL using Friis transmission equation, according to which the loss is proportional to the square of the distance and the square of the frequency.

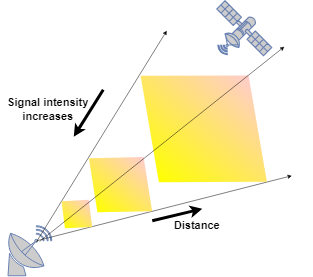
For an isotropic antenna that radiates equal power (P_t) in all directions, the power density is evenly distributed over the surface of a sphere with the isotropic antenna at its center. The intensity (I) of the signal is:

I = P_t / 4πd²,

where d is the distance between the transmitter and the receiver.

The power at the receiving antenna is P_r = I ✕ A_eff, where:

Substituting I and A_eff, P_r is:

$$P_r=\left(\frac{P_t}{4\pi d^2}\right)\left(\frac{{\lambda }^2}{4\pi }\right)$$,

Thus, FSPL (L_p) = $$\frac{P_t}{P_r}={\left(\frac{4\pi d}{\lambda }\right)}^2$$

Polarization Loss

Polarization mismatch between the transmitter and the receiver antennas leads to electromagnetic (EM) power loss. EM waves are characterized by electric and magnetic fields. A basic property of plane EM waves in free-space is that the directions of the electric and magnetic field vectors are orthogonal to their direction of propagation. EM wave polarization refers to the orientation of the electric field vector.

An antenna used to receive polarized electromagnetic waves achieves its maximum output power when the antenna polarization matches the polarization of the incident electromagnetic field. Otherwise, there is polarization loss, which can lead to a degree of signal coupling, in turn causing signal degradation. Polarization can be linear, circular, or elliptical, depending on the shape traced by the electric field vector. Polarization mismatch is characterized by a polarization loss factor, which describes the fraction of incident power that has the correct polarization for reception.

When signals traverse the ionosphere, an orthogonal component can emerge, leading to depolarization. You can quantify the impact of this phenomenon through these two metrics.

Raindrops contribute to depolarization as well. Ideally, a raindrop assumes a spherical shape to minimize the energy required for its cohesion. In reality, however, raindrops take on a flattened shape, resembling a spheroid with one axis longer than the others. The orientation of raindrops is randomized, influenced by factors such as wind, causing them to tilt. This tilt induces a rotation in the polarization of radio waves, which can be as much as 10 degrees. Such rotation poses a significant issue for systems using linear polarization, but it is less problematic for circular polarization, where the rotation merely adds to the existing spin of the wave.

Additionally, above the rain layer, there is often a layer of ice that can also lead to depolarization. Ice crystals typically form needle-like or plate-like shapes. Depolarization is negligible when these crystals are randomly oriented, but can occur when there is alignment.

Antenna Pointing Loss

Correct alignment between an Earth station and satellite antennas provides maximum gain. Misalignment can occur either at the satellite or at the Earth station. Satellite-based misalignment must be considered during the design of the satellite, but the Earth station-based misalignment is the antenna pointing loss, and it is typically less than 1 dB. Calculating antenna misalignment losses (AML) requires statistical data, so these values are an approximation based on real data observed in several ground stations.

Along with pointing loss, misalignment loss can also be due to misalignment in the direction of polarization. These losses are quite small. Thus, antenna misalignment loss is inclusive of pointing loss as well as polarization loss.

Antenna Noise Temperature

Antenna noise temperature represents the noise level an antenna produces in a given environment. This measurement is not the physical temperature of the antenna. The total noise temperature of the antenna depends mainly on sky noise (T_sky) and ground noise (T_gnd).

T_sky consists of two main components: atmospheric and background radiation. Because the upper atmosphere is an absorbing medium, the sky noise increases with elevation. T_gnd contributes dominantly to the antenna noise picked up through side lobes. Ground noise temperature increases with the decrease in elevation angle, as the side lobes intercepting the ground increase. A deep dish picks up less ground noise at lower elevations than a shallow one.

Direction of transmission also affects the antenna noise temperature. In the uplink transmission from a ground station antenna to space, the antenna temperature is quite low. For a satellite antenna transmitting downlink to the ground station antenna on Earth, the antenna temperature is around 290 K, which is the noise temperature of the Earth.

System noise temperature is the sum total of the thermal noise generated by all the components of the receiver chain, that is, the summation of antenna noise temperature and the noise contribution of the rest of the receiver system.

G/T

Antenna gain over noise temperature is a figure of merit to characterize the performance of an antenna, where G is the antenna gain, in decibels, at the receiver frequency, and T is the equivalent noise temperature of the receiving system in kelvin. T, referred to as system noise temperature, combines both antenna noise temperature and low noise amplifier (LNA) temperature.

The ratio of G and T is:

G/T = Antenna gain (dBi) – 10log(T)

HPA Power Backoff

When you feed HPA a multiple carrier signal as input, it amplifies the signal and, in the process, generates unwanted intermodulation (IM) products. When the amplifier operates in the linear region, these IM products do not interfere with the main signals. However, as the amplifier approaches the saturation point and enters the non-linear region of operation, the interference increases and these IM products start to interfere with the main signal, lowering the output signal quality. Introducing power backoff in an amplifier ensures the amplifier always operates at a power level below the saturation point in the linear region, even if the input power increases slightly. Typically, the amplifiers operate near the saturation point, as that is where efficiency is highest. You can apply power backoff at either the HPA input (known as input backoff or IBO) or at the HPA output (known as output backoff or OBO).

Rain Fade

Rainfall attenuates radio waves by scattering and absorbing energy from the wave. Rain attenuation increases with increasing frequency and is worse at Ku-band compared to C-band. Studies have shown that the rain attenuation for horizontal polarization is considerably greater than for vertical polarization. The link margin must compensate for a rain fade. In normal conditions, only oxygen and water vapour significantly contribute to absorption, but other atmospheric gases become a factor in very dry air conditions at frequencies above 70 GHz. International Telecommunication Union Recommendation (ITU-R) P.618 predicts the various atmospheric propagation parameters necessary in the planning of Earth-space networks. For details, see Earth-Space Propagation Losses.

Satellite Information

This includes information related to satellite aspects such as orbital position, received G/T obtained from satellite operators or G/T contour maps, and saturation flux density. This information helps effectively calculate a satellite link budget.

Carrier Information

This includes information such as user data rate in megabits per second (Mbps), type of modulation technique used, forward error correction (FEC) code rate, roll off factor, and bit error rate (BER). This information helps to analyze the link budget for a space-to-ground link and the inverse.

Satellite Carrier Spacing

The level of ACI is a function of several parameters: orbital separation between the desired and the interfering satellites, antenna side lobe performance of the interfering uplink Earth station, antenna side lobe performance of the receiving Earth station, and spectral power density of the carriers.

If the satellite carrier spacing is less than the recommended spacing, a spectral overlap from the adjacent carriers into the desired carrier is possible. At the demodulator, this unwanted energy acts as increased noise, reducing the effective carrier-to-noise ratio (CNR), C/N₀, and degrading the BER.