CPM Modulator Baseband

Modulate signal using CPM method

Libraries:
Communications Toolbox / Modulation / Digital Baseband Modulation / CPM

Description

The CPM Modulator Baseband block modulates an input signal using the continuous phase modulation (CPM) method. The output of the modulator is a baseband representation of the modulated signal.

For more information about the modulation and the filtering applied, see CPM Modulation and Pulse Shape Filtering.

Examples

expand all

View CPM Phase Tree Using Simulink

This example uses:

Open Model

The doc_cpm_phase_tree model uses the Eye Diagram block to view the in-phase and quadrature components, phase trajectory, phase tree, and instantaneous frequency of a CPM modulated signal.

Explore Model

A random integer signal is converted to bits and then CPM modulated. The CPM modulated signal values are converted from complex to magnitude, and angle, and then the phase is unwrapped.

Plot Eye Diagrams

Eye Diagram blocks are named to reflect the signal each displays. When you run the example, these Eye Diagram blocks show how the CPM signal changes over time:

Modulated Signal block — Displays the in-phase and quadrature signals. Double-click the block to open the scope. The modulated signal is easy to see in the eye diagram only when the Modulation index parameter in the CPM Modulator Baseband block is set to 1/2. For a modulation index value of 2/3, the modulation is more complex and the features of the modulated signal are difficult to decipher. Unwrapping the phase and plotting it is another way to illustrate these more complex CPM modulated signals.
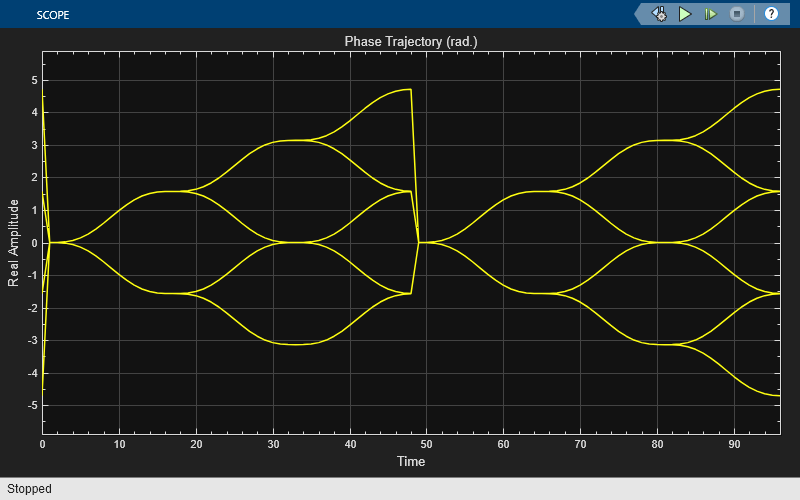
Phase Trajectory block — Displays the CPM phase. Double-click the block to open the scope. The Phase Trajectory block reveals that the signal phase is also difficult to view because it drifts with the data input to the modulator.
Phase Tree block — Displays the phase tree of the signal. The CPM phase is processed by a few simple blocks to make the CPM pulse shaping easier to view. This processing holds the phase at the beginning of the symbol interval and subtracts it from the signal. This zero-order hold resets the phase to zero every three symbols. The resulting plot shows the many phase trajectories that can be taken by the signal from any given symbol epoch.
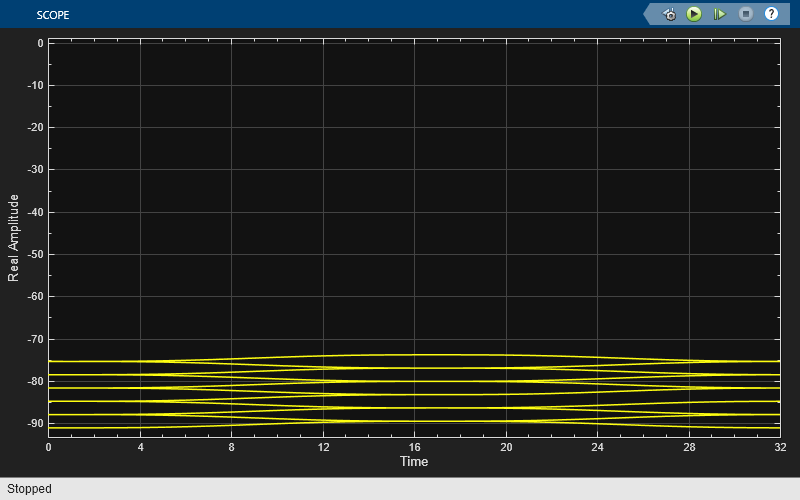
Instantaneous Frequency block — Displays the instantaneous frequency of the signal. The CPM phase is differentiated to produce the frequency deviation of the signal. Viewing the CPM frequency signal enables you to observe the frequency deviation qualitatively, as well as make quantitative observations, such as measuring peak frequency deviation.

Running the doc_cpm_phase_tree model opens and plots the phase tree and instantaneous frequency eye diagram plots.

Further Exploration

To learn more about the example, try changing the following parameters in the CPM Modulator Baseband block:

Change Pulse length to a value between 1 and 6.
Change Frequency pulse shape to one of the other settings, such as Rectangular or Gaussian.

You can observe the effect of changing these parameters on the phase tree and instantaneous frequency of the modulated signal.

Ports

Input

expand all

In — Input signal
scalar | column vector

Input signal, specified as a scalar or column vector.

When the Input type parameter is set to Integer, the block accepts odd integers in the range [ –(M–1), (M–1)]. M is the modulation order which is specified by the M-ary number parameter.

When the Input type parameter is set to Bit, the block accepts binary-valued inputs that represent integers. The block collects binary-valued signals into groups of k = log₂(M) bits. k is the number of bits per symbol and M is the modulation order. The input vector length must be an integer multiple of k. The block maps each group of k bits onto a symbol, as specified by the Symbol set ordering parameter. For each group of k bits, the block outputs one modulated symbol, oversampled by the Samples per symbol parameter value.

Supported Data Types

Double-precision floating point
Boolean is permitted when Input type is set to Bit
8-, 16-, and 32-bit signed integers are permitted when Input type is set to Integer

Data Types: double | Boolean | int8 | int16 | int32

Output

expand all

Out — Output signal
scalar | column vector

Output signal, returned as a scalar or column vector.

When the Input type parameter is set to Integer, the block outputs one modulated symbol for each input symbol.
When the Input type parameter is set to Bit, the block outputs one modulated symbol for each group of k bits.

In both cases, the modulated symbols are oversampled by the Samples per symbol parameter value.

Data Types: double | single

For more information on the processing rates, see Single-Rate Processing, and Multirate Processing.

Parameters

expand all

To edit block parameters interactively, use the Property Inspector. From the Simulink^® Toolstrip, on the Simulation tab, in the Prepare gallery, select Property Inspector.

M-ary number — Modulation order
`4` (default) | power of two scalar

Modulation order, specified as a power-of-two scalar. The modulation order, M = 2^k specifies the number of points in the signal constellation, where k is a positive integer indicating the number of bits per symbol.

Input type — Integer or group of bits input indicator
`Integer` (default) | `Bit`

Indicates whether the input consists of integers or groups of bits, specified as Integer or Bit.

Symbol set ordering — Symbol mapping
`Binary` (default) | `Gray`

Symbol mapping of bit inputs, specified as Binary or Gray.

Set this parameter to Binary to map symbols using binary-coded ordering.
Set this parameter to Gray to map symbols using Gray-coded ordering.

For more information, see Symbol Sets.

Dependencies

To enable this parameter, set Input type to Bit.

Modulation index — Modulation index {h_i}
`0.5` (default) | nonnegative scalar | column vector

Modulation index {h_i}, specified as a nonnegative scalar or column vector.

{h} represents a sequence of modulation indices. For more information, see CPM Modulation.

Frequency pulse shape — Type of pulse shaping
`Rectangular` (default) | `Raised Cosine` | `Spectral Raised Cosine` | `Gaussian` | `Tamed FM`

Type of pulse shaping used to smooth the phase transitions of the modulated signal, specified as Rectangular, Raised Cosine, Spectral Raised Cosine, Gaussian, or Tamed FM. For more information on the filtering options, see Pulse Shape Filtering.

Main lobe pulse duration (symbol intervals) — Main lobe duration
`1` (default) | positive integer

Main lobe duration of the largest lobe in the spectral raised cosine pulse, specified as a positive integer representing the number of symbol intervals used by the modulator to pulse-shape the modulated signal.

Dependencies

To enable this parameter, set Frequency pulse shape to Spectral Raised Cosine.

Rolloff — Rolloff factor of spectral raised cosine pulse shape
`0.2` (default) | nonnegative scalar

Rolloff factor of the spectral raised cosine pulse, specified as a scalar from 0 to 1.

Dependencies

To enable this parameter, set Frequency pulse shape to Spectral Raised Cosine.

BT product — Product of bandwidth and symbol time of Gaussian pulse shape
`0.3` (default) | positive scalar

Product of the bandwidth and symbol time of the Gaussian pulse shape, specified as a positive scalar. Use BT product to reduce the bandwidth, at the expense of increased intersymbol interference.

Dependencies

To enable this parameter, set Frequency pulse shape to Gaussian.

Pulse length (symbol intervals) — Length of frequency pulse shape
`1` (default) | positive integer

Length of the frequency pulse shape in symbol intervals, specified as a positive integer. For more information on the frequency pulse length, refer to LT in Pulse Shape Filtering.

Symbol prehistory — Data symbols used before the start of simulation
`1` (default) | scalar | vector

Data symbols used before the start of simulation, specified as scalar or vector with odd integer elements in the range [– (M-ary number – 1), (M-ary number – 1)]. The Symbol prehistory parameter defines the data symbols used by the modulator prior to the first call of the block, in reverse chronological order.

A scalar value expands to a vector of length L_P – 1. L_P represents the pulse length, which is specified by the Pulse length (symbol intervals) parameter.
For a vector, the length must be L_P – 1.

Phase offset (rad) — Initial phase offset
`0` (default) | scalar

Initial phase offset in radians of the modulated waveform, specified as a scalar.

Samples per symbol — Symbol sampling rate
`8` (default) | positive scalar

Symbol sampling rate, specified as a positive scalar. This parameter represents the number of samples output for each integer or binary word input. For all nonbinary schemes, as defined by the pulse shapes, this value must be greater than 1.

For more information, see Signal Upsampling and Rate Changes.

Rate options — Block processing rate
`Enforce single-rate processing` (default) | `Allow multirate processing`

Block processing rate, specified as one of these options:

Enforce single-rate processing — The input and output signals have the same sample time. The block implements the rate change by making a size change at the output when compared to the input. The output width equals the product of the number of symbols and the Samples per symbol parameter value.
Allow multirate processing — The input and output signals have different sample times. The output sample time equals the symbol period divided by the Samples per symbol parameter value.

Output data type — Output data type
`double` (default) | `single`

Output data type, specified as double or single.

Block Characteristics

Data Types	`Boolean` \| `double` \| `integer` \| `single`
Multidimensional Signals	`no`
Variable-Size Signals	`no`

More About

expand all

CPM Modulation

The output of the modulator is a baseband representation of the modulated signal:

$\begin{array}{l} s (t) = \exp [j 2 π \sum_{i = 0}^{n} α_{i} h_{i} q (t - i T)], and \\ n T < t < (n + 1) T . \end{array}$

where:

{α_i} is a sequence of M-ary data symbols selected from the alphabet ±1, ±3, ±(M–1).
M must have the form 2^k for some positive integer k, where M is the modulation order and specifies the size of the symbol alphabet.
{h_i} is a sequence of modulation indices. h_i moves cyclically through a set of indices {h₀, h₁, h₂, ..., h_H-1}. When H=1, only one modulation index exists, h₀, which is denoted as h.

h_i specifies the modulation index. When h_i varies from interval to interval, the block operates in multi-h. To ensure a finite number of phase states, h_i must be a rational number.

Pulse Shape Filtering

The CPM method uses pulse shaping to smooth the phase transitions of the modulated signal. The function q(t) is the phase response obtained from the frequency pulse, g(t), through this relation: $q (t) = \int_{- \infty}^{t} g (t) d t$ .

The specified frequency pulse shape corresponds to these pulse shape expressions for g(t).

Pulse Shape	Expression
Rectangular	$g (t) = {\begin{matrix} \frac{1}{2 L T}, & 0 \leq t \leq L T \\ 0 & otherwise \end{matrix}$
Raised cosine	$g (t) = {\begin{matrix} \frac{1}{2 L T} [1 - \cos (\frac{2 π t}{L T})], & 0 \leq t \leq L T \\ 0 & otherwise \end{matrix}$
Spectral raised cosine	$g (t) = \frac{1}{L_{main} T} \frac{\sin (\frac{2 π t}{L_{main} T})}{\frac{2 π t}{L_{main} T}} \frac{\cos (β \frac{2 π t}{L_{main} T})}{1 - {(\frac{4 β}{L_{main} T} t)}^{2}}, 0 \leq β \leq 1$
Gaussian	$\begin{matrix} g (t) = \frac{1}{2 T} {Q [2 π B_{b} \frac{t - \frac{T}{2}}{\sqrt{\ln 2}}] - Q [2 π B_{b} \frac{t + \frac{T}{2}}{\sqrt{\ln 2}}]}, where \\ Q (t) = \int_{t}^{\infty} \frac{1}{\sqrt{2 π}} e^{- τ^{2} / 2} d τ \end{matrix}$
Tamed FM (tamed frequency modulation)	$\begin{array}{l} g (t) = \frac{1}{8} [g_{0} (t - T) + 2 g_{0} (t) + g_{0} (t + T)], where \\ g_{0} (t) \approx \frac{1}{T} [\frac{\sin (\frac{π t}{T})}{\frac{π t}{T}} - \frac{π^{2}}{24} \frac{2 \sin (\frac{π t}{T}) - \frac{2 π t}{T} \cos (\frac{π t}{T}) - {(\frac{π t}{T})}^{2} \sin (\frac{π t}{T})}{{(\frac{π t}{T})}^{3}}] \end{array}$

L_main is the main lobe pulse duration in symbol intervals.
β is the roll-off factor of the spectral raised cosine.
B_b is the product of the bandwidth and the Gaussian pulse.
The duration of the pulse, LT, is the pulse length in symbol intervals. As defined by the expressions, the spectral raised cosine, Gaussian, and tamed FM pulse shapes have infinite length. For all practical purposes, LT specifies the truncated finite length.
T is the symbol durations.
Q(t) is the complementary cumulative distribution function.

For more information on pulse shape filtering, see [1].

Symbol Sets

In binary input mode, the block processing follows this procedure.

Divide the input bits into k-length bit words and map each to an integer,L, in the range [0, M – 1]. Where k = log2(M) and M is the modulation order specified by the M-ary number parameter. The binary word mapping options are binary-coded ordering or Gray-coded ordering, as specified by the Symbol set ordering parameter.
Map each integer L to signed integers, as 2L–(M–1).
Proceed with modulation processing as in the integer input mode.

Single-Rate Processing

In single-rate processing mode, the input and output signals have the same port sample time. In this mode, the input to the block can be multiple symbols. The block implicitly implements the rate change by making a size change at the output when compared to the input.

When you set Input type to Integer, the input can be a scalar or a column vector with the length equal to the number of input symbols.
When you set Input type to Bit, the input width must be an integer multiple of the number of bits per symbol.

The output width equals N_Sym × N_SPS, where N_Sym is the number of symbols in the frame and N_SPS is the number of samples per symbol.

Multirate Processing

In multirate processing mode, the input and output signals have different port sample times. In this mode, the input to the block must be one symbol.

When you set Input type to Integer, the input must be a scalar.
When you set Input type to Bit, the input width must equal the number of bits per symbol.

The output sample time equals T_Sym / N_SPS, where T_Sym is the symbol period and N_SPS is the number of samples per symbol.

References

[1] Anderson, John B., Tor Aulin, and Carl-Erik Sundberg. Digital Phase Modulation. New York: Plenum Press, 1986.

Extended Capabilities

C/C++ Code Generation
Generate C and C++ code using Simulink® Coder™.

Version History

Introduced before R2006a

CPM Modulator Baseband

Description

Examples

View CPM Phase Tree Using Simulink

Ports

Input

In — Input signal scalar | column vector

Supported Data Types

Output

Out — Output signal scalar | column vector

Parameters

M-ary number — Modulation order 4 (default) | power of two scalar

Input type — Integer or group of bits input indicator Integer (default) | Bit

Symbol set ordering — Symbol mapping Binary (default) | Gray

Dependencies

Modulation index — Modulation index {hi} 0.5 (default) | nonnegative scalar | column vector

Frequency pulse shape — Type of pulse shaping Rectangular (default) | Raised Cosine | Spectral Raised Cosine | Gaussian | Tamed FM

Main lobe pulse duration (symbol intervals) — Main lobe duration 1 (default) | positive integer

Dependencies

Rolloff — Rolloff factor of spectral raised cosine pulse shape 0.2 (default) | nonnegative scalar

Dependencies

BT product — Product of bandwidth and symbol time of Gaussian pulse shape 0.3 (default) | positive scalar

Dependencies

Pulse length (symbol intervals) — Length of frequency pulse shape 1 (default) | positive integer

Symbol prehistory — Data symbols used before the start of simulation 1 (default) | scalar | vector

Phase offset (rad) — Initial phase offset 0 (default) | scalar

Samples per symbol — Symbol sampling rate 8 (default) | positive scalar

Rate options — Block processing rate Enforce single-rate processing (default) | Allow multirate processing

Output data type — Output data type double (default) | single

Block Characteristics

More About

CPM Modulation

Pulse Shape Filtering

Symbol Sets

Single-Rate Processing

Multirate Processing

References

Extended Capabilities

C/C++ Code Generation Generate C and C++ code using Simulink® Coder™.

Version History

See Also

Blocks

Objects

Topics

In — Input signal
scalar | column vector

Out — Output signal
scalar | column vector

M-ary number — Modulation order
`4` (default) | power of two scalar

Input type — Integer or group of bits input indicator
`Integer` (default) | `Bit`

Symbol set ordering — Symbol mapping
`Binary` (default) | `Gray`

Modulation index — Modulation index {h_i}
`0.5` (default) | nonnegative scalar | column vector

Frequency pulse shape — Type of pulse shaping
`Rectangular` (default) | `Raised Cosine` | `Spectral Raised Cosine` | `Gaussian` | `Tamed FM`

Main lobe pulse duration (symbol intervals) — Main lobe duration
`1` (default) | positive integer

Rolloff — Rolloff factor of spectral raised cosine pulse shape
`0.2` (default) | nonnegative scalar

BT product — Product of bandwidth and symbol time of Gaussian pulse shape
`0.3` (default) | positive scalar

Pulse length (symbol intervals) — Length of frequency pulse shape
`1` (default) | positive integer

Symbol prehistory — Data symbols used before the start of simulation
`1` (default) | scalar | vector

Phase offset (rad) — Initial phase offset
`0` (default) | scalar

Samples per symbol — Symbol sampling rate
`8` (default) | positive scalar

Rate options — Block processing rate
`Enforce single-rate processing` (default) | `Allow multirate processing`

Output data type — Output data type
`double` (default) | `single`

C/C++ Code Generation
Generate C and C++ code using Simulink® Coder™.