shortestpath
Shortest path between two single nodes
Syntax
Description
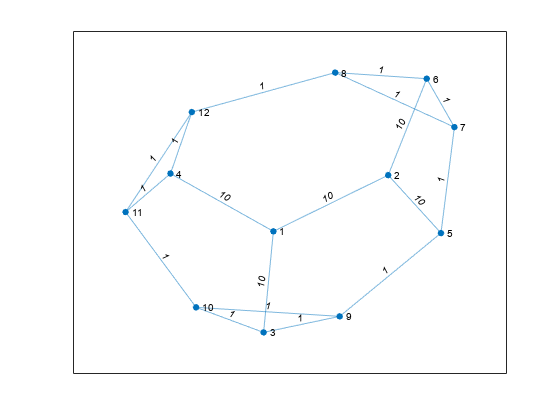
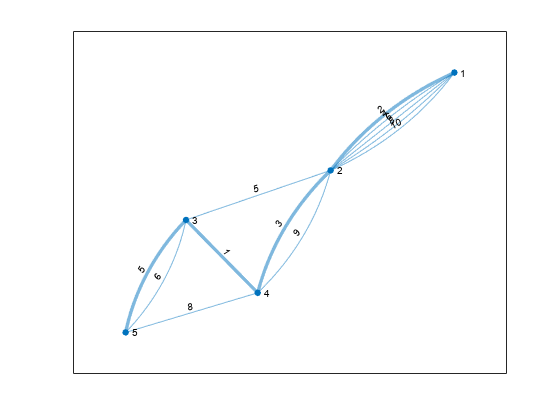
P = shortestpath(G,s,t)s and ending
at target node t. If the graph is weighted (that is,
G.Edges contains a variable Weight), then
those weights are used as the distances along the edges in the graph. Otherwise, all
edge distances are taken to be 1.
Examples
Input Arguments
Output Arguments
Tips
The
shortestpath,shortestpathtree, anddistancesfunctions do not support undirected graphs with negative edge weights, or more generally any graph containing a negative cycle, for these reasons:A negative cycle is a path that leads from a node back to itself, with the sum of the edge weights on the path being negative. If a negative cycle is on a path between two nodes, then no shortest path exists between the nodes, since a shorter path can always be found by traversing the negative cycle.
A single negative edge weight in an undirected graph creates a negative cycle.
Extended Capabilities
Version History
Introduced in R2015b
See Also
shortestpathtree | distances | nearest | graph | digraph