ctf2zp

Convert cascaded transfer functions to zero-pole-gain form

Since R2024b

Syntax

[z,p] = ctf2zp(B,A)

[z,p] = ctf2zp(B,A,g)

[___,k] = ctf2zp(___)

Description

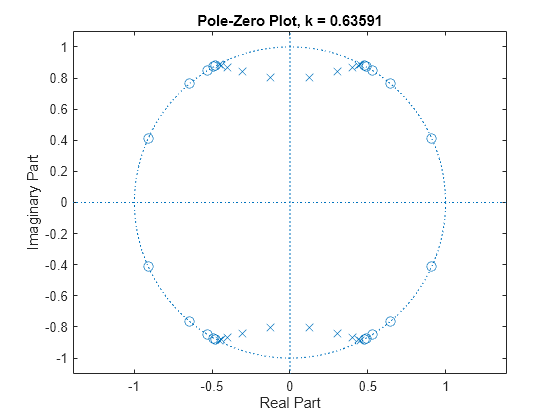
[z,p] = ctf2zp(B,A) computes the zeros z and poles p of a system represented as Cascaded Transfer Functions (CTF) with numerator coefficients B and denominator coefficients A.

example

[z,p] = ctf2zp(B,A,g) specifies the scale values, g, across filter sections.

[___,k] = ctf2zp(___) returns the scalar gain k of the system. Use this syntax with any of the input or output arguments in previous syntaxes.

example

Examples

collapse all

Zeros and Poles from Two-Section Digital Filter

Open Live Script

Specify a two-section digital filter with a transfer function $H (z)$ in the CTF format. Plot the 1024-point frequency response of the filter.

$H (z) = (0.1607 + 0.2414 z^{- 1} + 0.4689 z^{- 2}) \times \frac{0.1607 + 0.0828 z^{- 1} + 0.0551 z^{- 2}}{1 - 1.1940 z^{- 1} + 0.4360 z^{- 2}}$

B = [0.1607    0.2414    0.4689
     0.1607    0.0828    0.0551];
A = [     1         0         0;
          1   -1.1940    0.4360];
freqz(B,A,1024,"ctf")

$Figure contains 2 axes objects. Axes object 1 with title Phase, xlabel Normalized Frequency (\times\pi rad/sample), ylabel Phase (degrees) contains an object of type line. Axes object 2 with title Magnitude, xlabel Normalized Frequency (\times\pi rad/sample), ylabel Magnitude (dB) contains an object of type line.$

Compute the zeros and poles of the transfer function.

[z,p] = ctf2zp(B,A)

z = 4×1 complex

  -0.7511 + 1.5342i
  -0.7511 - 1.5342i
  -0.2576 + 0.5258i
  -0.2576 - 0.5258i

p = 4×1 complex

   0.0000 + 0.0000i
   0.0000 + 0.0000i
   0.5970 + 0.2821i
   0.5970 - 0.2821i

Zero-Pole-Gain from Cascaded Transfer Function

Open Live Script

Create a 10th-order bandpass digital elliptic filter in the CTF form.

[B,A,gS] = ellip(10,0.1,60,[0.35 0.65],"ctf")

B = 5×5

    1.0000   -0.0000   -1.3205    0.0000    1.0000
    1.0000    0.0000    0.3308   -0.0000    1.0000
    1.0000   -0.0000    0.8670   -0.0000    1.0000
    1.0000    0.0000    1.0340    0.0000    1.0000
    1.0000    0.0000    1.0853   -0.0000    1.0000

A = 5×5

    1.0000   -0.0000    1.2677   -0.0000    0.4407
    1.0000    0.0000    1.2212    0.0000    0.6374
    1.0000   -0.0000    1.1779   -0.0000    0.8251
    1.0000    0.0000    1.1571    0.0000    0.9300
    1.0000   -0.0000    1.1561   -0.0000    0.9818

gS = 
0.0053

Specify scale values. Because the filter has five fourth-order sections, the vector of scale values must have six elements.

g = [1:size(B,1) gS]

g = 1×6

    1.0000    2.0000    3.0000    4.0000    5.0000    0.0053

Get the zero-pole decomposition of the filter. Plot the zeros and poles in the z-plane.

[z,p,k] = ctf2zp(B,A,g);
zplane(z,p)
title("Pole-Zero Plot, k = "+k)

Figure contains an axes object. The axes object with title Pole-Zero Plot, k = 0.63591, xlabel Real Part, ylabel Imaginary Part contains 3 objects of type line. One or more of the lines displays its values using only markers

Input Arguments

collapse all

`B,A` — Cascaded transfer function (CTF) coefficients
scalars | vectors | matrices

Since R2024b

Cascaded transfer function (CTF) coefficients, specified as scalars, vectors, or matrices. B and A list the numerator and denominator coefficients of the cascaded transfer function, respectively.

B must be of size L-by-(m + 1) and A must be of size L-by-(n + 1), where:

L represents the number of filter sections.
m represents the order of the filter numerators.
n represents the order of the filter denominators.

For more information about the cascaded transfer function format and coefficient matrices, see Specify Digital Filters in CTF Format.

Note

If any element of A(:,1) is not equal to 1, then ctf2zp normalizes the filter coefficients by A(:,1). In this case, A(:,1) must be nonzero.

Data Types: double | single
Complex Number Support: Yes

`g` — Scale values
`1` (default) | scalar | vector

Since R2024b

Scale values, specified as a real-valued scalar or as a real-valued vector with L + 1 elements, where L is the number of CTF sections. The scale values represent the distribution of the filter gain across the sections of the cascaded filter representation.

The ctf2zp function applies a gain to the filter sections using the scaleFilterSections function depending on how you specify g:

Scalar — The function distributes the gain uniformly across all filter sections.
Vector — The function applies the first L gain values to the corresponding filter sections and distributes the last gain value uniformly across all filter sections.

Data Types: double | single

Output Arguments

collapse all

`z,p` — System zeros and poles
column vectors

System zeros and poles, returned as column vectors.

The vectors z and p contain the n zeros and m poles of the transfer function H(z), respectively.

$H (z) = k \frac{(z - z_{1}) (z - z_{2}) \dots (z - z_{n})}{(z - p_{1}) (z - p_{2}) \dots (z - p_{m})} .$

`k` — System gain
scalar

System gain, returned as a scalar.

The scalar k represents the gain of the transfer function H(z).

$H (z) = k \frac{(z - z_{1}) (z - z_{2}) \dots (z - z_{n})}{(z - p_{1}) (z - p_{2}) \dots (z - p_{m})} .$

More About

collapse all

Cascaded Transfer Functions

Partitioning an IIR digital filter into cascaded sections improves its numerical stability and reduces its susceptibility to coefficient quantization errors. The cascaded form of a transfer function H(z) in terms of the L transfer functions H₁(z), H₂(z), …, H_L(z) is

$H (z) = \prod_{l = 1}^{L} H_{l} (z) = H_{1} (z) \times H_{2} (z) \times \dots \times H_{L} (z) .$

Specify Digital Filters in CTF Format

You can specify digital filters in the CTF format for analysis, visualization, and signal filtering. Specify a filter by listing its coefficients B and A. You can also include the filter scaling gain across sections by specifying a scalar or vector g.

Filter Coefficients

When you specify the coefficients as L-row matrices,

$B = [\begin{matrix} b_{11} & b_{12} & \dots & b_{1, m + 1} \\ b_{21} & b_{22} & \dots & b_{2, m + 1} \\ ⋮ & ⋮ & ⋱ & ⋮ \\ b_{L 1} & b_{L 2} & \dots & b_{L, m + 1} \end{matrix}], A = [\begin{matrix} a_{11} & a_{12} & \dots & a_{1, n + 1} \\ a_{21} & a_{22} & \dots & a_{2, n + 1} \\ ⋮ & ⋮ & ⋱ & ⋮ \\ a_{L 1} & a_{L 2} & \dots & a_{L, n + 1} \end{matrix}],$

it is assumed that you have specified the filter as a sequence of L cascaded transfer functions, such that the full transfer function of the filter is

$H (z) = \frac{b_{11} + b_{12} z^{- 1} + \dots + b_{1, m + 1} z^{- m}}{a_{11} + a_{12} z^{- 1} + \dots + a_{1, n + 1} z^{- n}} \times \frac{b_{21} + b_{22} z^{- 1} + \dots + b_{2, m + 1} z^{- m}}{a_{21} + a_{22} z^{- 1} + \dots + a_{2, n + 1} z^{- n}} \times \dots \times \frac{b_{L 1} + b_{L 2} z^{- 1} + \dots + b_{L, m + 1} z^{- m}}{a_{L 1} + a_{L 2} z^{- 1} + \dots + a_{L, n + 1} z^{- n}},$

where m ≥ 0 is the numerator order of the filter and n ≥ 0 is the denominator order.

If you specify both B and A as vectors, it is assumed that the underlying system is a one-section IIR filter (L = 1), with B representing the numerator of the transfer function and A representing its denominator.
If B is scalar, it is assumed that the filter is a cascade of all-pole IIR filters with each section having an overall system gain equal to B.
If A is scalar, it is assumed that the filter is a cascade of FIR filters with each section having an overall system gain equal to 1/A.

Note

To convert second-order section matrices to cascaded transfer functions, use the sos2ctf function.
To convert a zero-pole-gain filter representation to cascaded transfer functions, use the zp2ctf function.

Coefficients and Gain

If you have an overall scaling gain or multiple scaling gains factored out from the coefficient values, you can specify the coefficients and gain as a cell array of the form {B,A,g}. Scaling filter sections is especially important when you work with fixed-point arithmetic to ensure that the output of each filter section has similar amplitude levels, which helps avoid inaccuracies in the filter response due to limited numeric precision.

The gain can be a scalar overall gain or a vector of section gains.

If the gain is scalar, the value applies uniformly to all the cascade filter sections.
If the gain is a vector, it must have one more element than the number of filter sections L in the cascade. Each of the first L scale values applies to the corresponding filter section, and the last value applies uniformly to all the cascade filter sections.

If you specify the coefficient matrices and gain vector as

it is assumed that the transfer function of the filter system is

$H (z) = g_{S} (g_{1} \frac{b_{11} + b_{12} z^{- 1} + \dots + b_{1, m + 1} z^{- m}}{a_{11} + a_{12} z^{- 1} + \dots + a_{1, n + 1} z^{- n}} \times g_{2} \frac{b_{21} + b_{22} z^{- 1} + \dots + b_{2, m + 1} z^{- m}}{a_{21} + a_{22} z^{- 1} + \dots + a_{2, n + 1} z^{- n}} \times \dots \times g_{L} \frac{b_{L 1} + b_{L 2} z^{- 1} + \dots + b_{L, m + 1} z^{- m}}{a_{L 1} + a_{L 2} z^{- 1} + \dots + a_{L, n + 1} z^{- n}}) .$

Tips

You can obtain filters in CTF format, including the scaling gain. Use the outputs of digital IIR filter design functions, such as butter, cheby1, cheby2, and ellip. Specify the "ctf" filter-type argument in these functions and specify to return B, A, and g to get the scale values. (since R2024b)

References

[1] Lyons, Richard G. Understanding Digital Signal Processing. Upper Saddle River, NJ: Prentice Hall, 2004.

Extended Capabilities

C/C++ Code Generation
Generate C and C++ code using MATLAB® Coder™.

Version History

Introduced in R2024b

ctf2zp

Syntax

Description

Examples

Zeros and Poles from Two-Section Digital Filter

Zero-Pole-Gain from Cascaded Transfer Function

Input Arguments

`B,A` — Cascaded transfer function (CTF) coefficients
scalars | vectors | matrices

`g` — Scale values
`1` (default) | scalar | vector

Output Arguments

`z,p` — System zeros and poles
column vectors

`k` — System gain
scalar

More About

Cascaded Transfer Functions

Specify Digital Filters in CTF Format

Tips

References

Extended Capabilities

C/C++ Code Generation
Generate C and C++ code using MATLAB® Coder™.

Version History

See Also

Apps

Functions

ctf2zp

Syntax

Description

Examples

Zeros and Poles from Two-Section Digital Filter

Zero-Pole-Gain from Cascaded Transfer Function

Input Arguments

B,A — Cascaded transfer function (CTF) coefficients scalars | vectors | matrices

g — Scale values 1 (default) | scalar | vector

Output Arguments

z,p — System zeros and poles column vectors

k — System gain scalar

More About

Cascaded Transfer Functions

Specify Digital Filters in CTF Format

Tips

References

Extended Capabilities

C/C++ Code Generation Generate C and C++ code using MATLAB® Coder™.

Version History

See Also

Apps

Functions

`B,A` — Cascaded transfer function (CTF) coefficients
scalars | vectors | matrices

`g` — Scale values
`1` (default) | scalar | vector

`z,p` — System zeros and poles
column vectors

`k` — System gain
scalar

C/C++ Code Generation
Generate C and C++ code using MATLAB® Coder™.