Time-Frequency Analysis

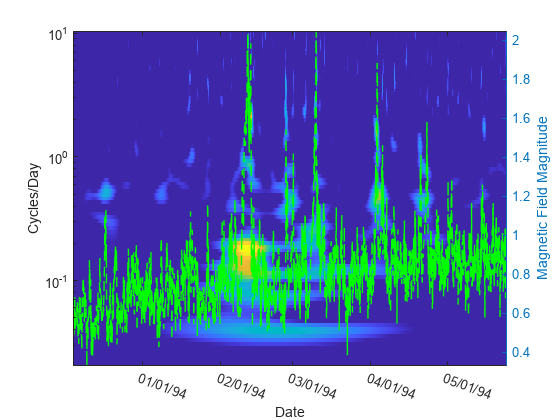
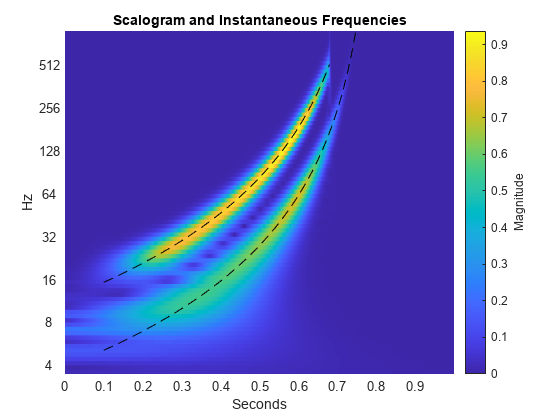
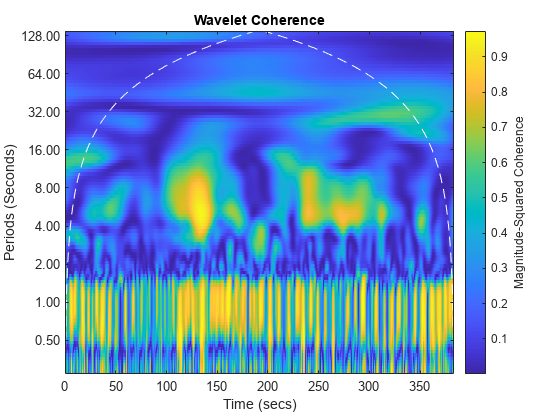
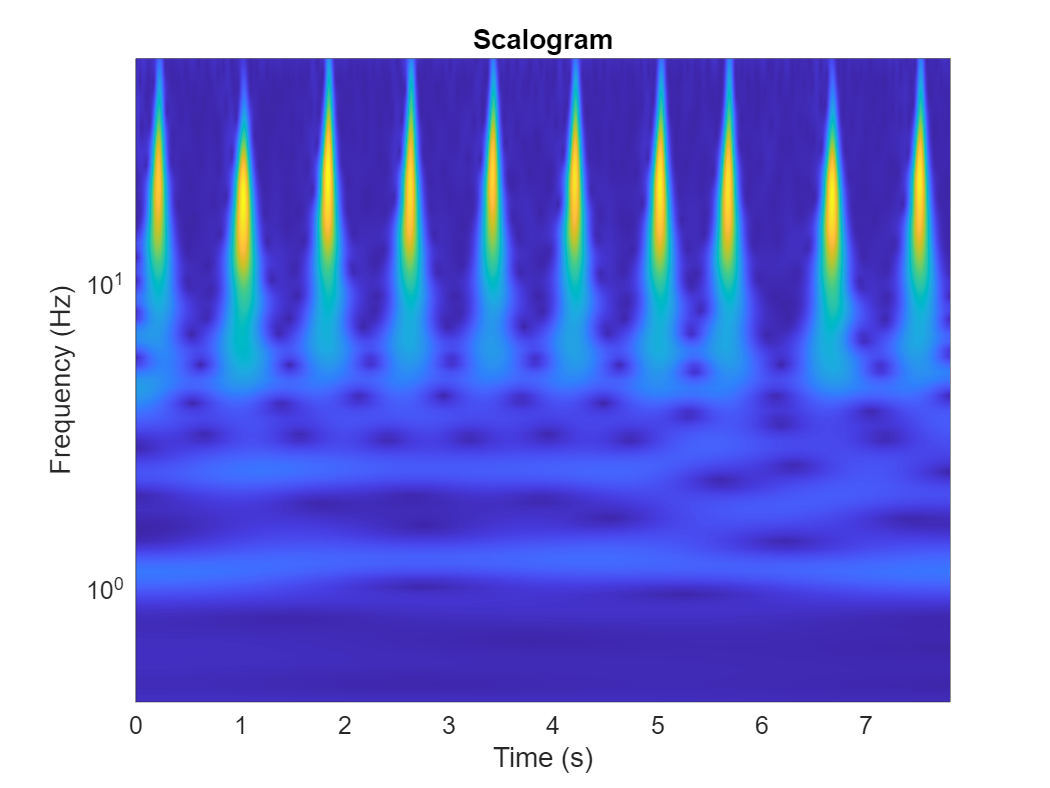
You can use the continuous wavelet transform (CWT) to analyze how the frequency content of a signal changes over time. You can perform adaptive time-frequency analysis using nonstationary Gabor frames with the constant-Q transform (CQT). For two signals, wavelet coherence reveals common time-varying patterns. You can perform data-adaptive time-frequency analysis of nonlinear and nonstationary processes. For images, continuous wavelet analysis shows how the frequency content of an image varies across the image and helps to reveal patterns in a noisy image. To obtain sharper resolution and extract oscillating modes from a signal, you can use wavelet synchrosqueezing.

Use Wavelet Toolbox™ to perform time-frequency analysis of signals and images. With the CQT, you can differentially sample the bandwidth, using more frequency samples for broader band components and less frequency samples for narrow band components. You can use the CWT to obtain the wavelet coherence between two signals. You can decompose a nonlinear or nonstationary process into its intrinsic modes of oscillation. You can also reconstruct time-frequency localized approximations to signals. You can create a CWT filter bank with specific frequency or period ranges, and efficiently apply the filter bank to multiple signals. You can visualize the wavelets in time and frequency.